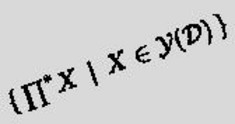Mathematical group hierarchies

A group is a simple mathematical structure that describes symmetries, like those of a geometric figure, a pattern on a wallpaper, or a crystal. For example, the cyclic group C₃ describes the three states of rotating an equilateral triangle; the cyclic group C₄ describes the four states of rotating a square; the Klein four-group K₄ describes the four states of a rectangle that can be rotated by 180 degrees or flipped. There exists one group of order three (C₃), but two groups of order four (C₄ and K₄). For the number N(k) of groups (up to isomorphism) of order k it means N(3)=1 and N(4)=2. But to understand N(k) for general k is puzzling. Particularly many groups exist of order a power of two, like N(64) = 267, or, for example, more than 99% of groups of order less than 2000 are of order k=1024. A hint to the fundamental interest in this question is the fact that it is the very first sequence in the well-known "Online encycolpedia of integer sequences":
oeis.org/A000001

An immediate upper bound for N(k) is 2^k, since no more Cayley tables can exist. This bound is not helpful for finite groups, but it is sharp for infinite groups: There exist N(k) = 2^k isomorphy classes of infinite groups of cardinality k. In "gathering" such infinitudes of groups it can be relevant to consider a big "universal" group that contains many groups embedded in it. So the question arises if the sharpness of N(k) = 2^k for infinite groups depends on this trick. Macintyre and Shelah showed in 1976 that for regular cardinalities the trick is unneccessary: there are 2^k pairwise non-embeddable groups of regular cardinality k. The remaining case of singular cardinalities was open for decades. The question is solved in 2025 by Gerald Kuba from BOKU University, Institute of Mathematics: For every infinite cardinal k there exist 2^k pairwise non-embeddable groups of cardinality k.
arxiv.org/abs/2401.17962